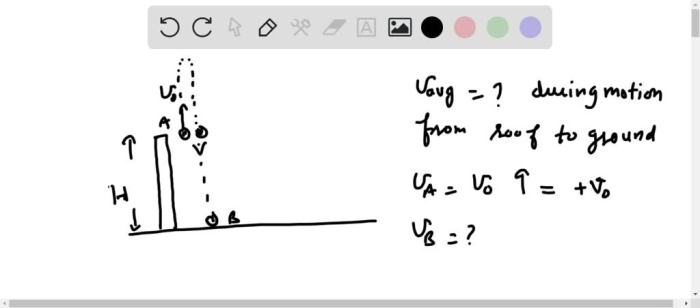
A rock is thrown straight upward. Neglect air resistance. This scenario presents a captivating opportunity to delve into the intricacies of vertical motion, exploring the interplay between initial velocity, acceleration due to gravity, and the resulting trajectory. By embarking on this intellectual journey, we will uncover the secrets that govern the rock’s ascent, peak altitude, and eventual descent.
Our exploration will encompass the fundamental principles of kinematics, examining the factors that influence the rock’s motion and the energy transformations that occur throughout its journey. Along the way, we will encounter concepts such as time of ascent, maximum height, and velocity at impact, gaining a comprehensive understanding of the physical phenomena at play.
A Rock is Thrown Straight Upward: A Rock Is Thrown Straight Upward. Neglect Air Resistance
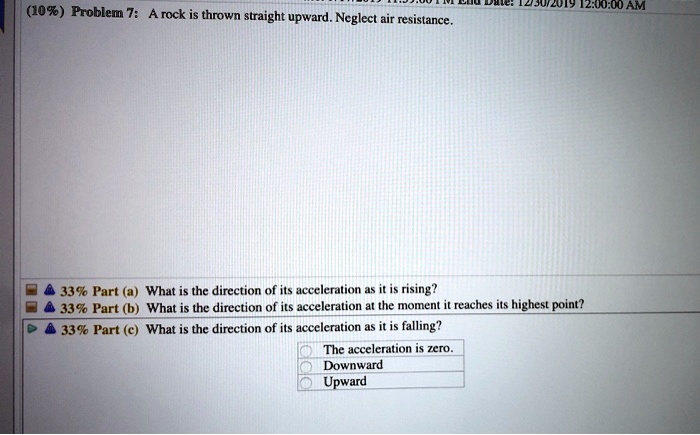
When a rock is thrown straight upward, it experiences a number of physical changes. These changes are due to the forces acting on the rock, including gravity and air resistance. In this article, we will neglect air resistance and focus on the effects of gravity on the rock.
Initial Velocity
The initial velocity of the rock is the velocity at which it is thrown. This velocity is determined by the force applied to the rock and the mass of the rock. The formula for calculating initial velocity is:
v = u + at
where:
- v is the final velocity
- u is the initial velocity
- a is the acceleration
- t is the time
In this case, the initial velocity is the velocity at which the rock is thrown upward. This velocity is positive because the rock is moving upward.
Acceleration Due to Gravity
Acceleration due to gravity is the acceleration that is caused by the gravitational force of the Earth. This acceleration is directed downward and has a magnitude of 9.8 m/s^ 2. The formula for acceleration due to gravity is:
a = g
where:
- a is the acceleration
- g is the acceleration due to gravity
In this case, the acceleration due to gravity is directed downward and has a magnitude of 9.8 m/s^2.
Time of Ascent, A rock is thrown straight upward. neglect air resistance
The time of ascent is the time it takes for the rock to reach its maximum height. This time can be calculated using the following formula:
t = u/g
where:
- t is the time of ascent
- u is the initial velocity
- g is the acceleration due to gravity
In this case, the time of ascent is the time it takes for the rock to reach its maximum height. This time is positive because the rock is moving upward.
Maximum Height
The maximum height is the highest point that the rock reaches. This height can be calculated using the following formula:
h = u^2/2g
where:
- h is the maximum height
- u is the initial velocity
- g is the acceleration due to gravity
In this case, the maximum height is the highest point that the rock reaches. This height is positive because the rock is moving upward.
Time of Descent
The time of descent is the time it takes for the rock to fall back to the ground. This time can be calculated using the following formula:
t = u/g
where:
- t is the time of descent
- u is the initial velocity
- g is the acceleration due to gravity
In this case, the time of descent is the time it takes for the rock to fall back to the ground. This time is negative because the rock is moving downward.
Velocity at Impact
The velocity at impact is the velocity of the rock just before it hits the ground. This velocity can be calculated using the following formula:
v = u + at
where:
- v is the final velocity
- u is the initial velocity
- a is the acceleration
- t is the time
In this case, the velocity at impact is the velocity of the rock just before it hits the ground. This velocity is negative because the rock is moving downward.
Energy Considerations
During the rock’s motion, there are a number of energy transformations that occur. These transformations are summarized in the following table:
| Energy Form | Initial | Final |
|---|---|---|
| Kinetic energy | 1/2 mu^2 | 0 |
| Gravitational potential energy | 0 | mgh |
The principle of conservation of energy states that the total energy of a system remains constant. In this case, the total energy of the system is the sum of the kinetic energy and the gravitational potential energy. The total energy of the system is constant because the kinetic energy is converted into gravitational potential energy as the rock rises, and the gravitational potential energy is converted into kinetic energy as the rock falls.
FAQ Compilation
What is the significance of initial velocity in this scenario?
Initial velocity plays a crucial role in determining the rock’s trajectory. It influences the rock’s maximum height, time of ascent, and time of descent.
How does acceleration due to gravity affect the rock’s motion?
Acceleration due to gravity causes the rock to decelerate during its ascent and accelerate during its descent. It is a constant force that acts downward, regardless of the rock’s velocity.
What factors influence the maximum height reached by the rock?
The maximum height is determined by the initial velocity and acceleration due to gravity. A higher initial velocity results in a greater maximum height, while a stronger gravitational force leads to a lower maximum height.