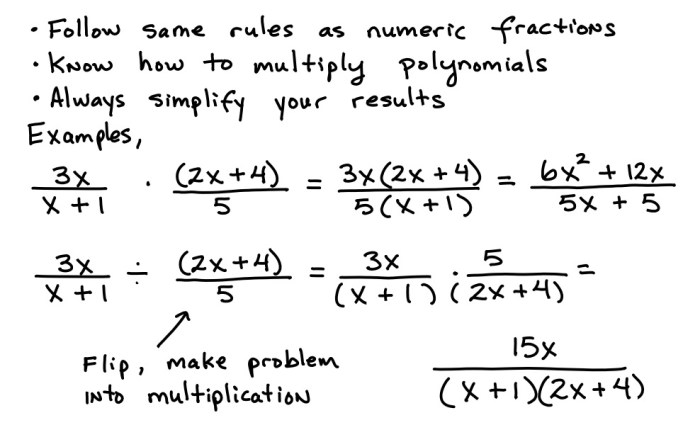
Multiplying Polynomials Algebra 2 Worksheet provides an in-depth exploration of the techniques and applications of polynomial multiplication. This worksheet serves as a valuable resource for students seeking to master this fundamental algebraic operation.
Through a combination of clear explanations, illustrative examples, and engaging exercises, this worksheet empowers learners to develop a comprehensive understanding of multiplying polynomials. It covers various methods, special products, and real-world applications, ensuring a thorough grasp of this essential concept.
Multiplying Polynomials Algebra 2: Multiplying Polynomials Algebra 2 Worksheet
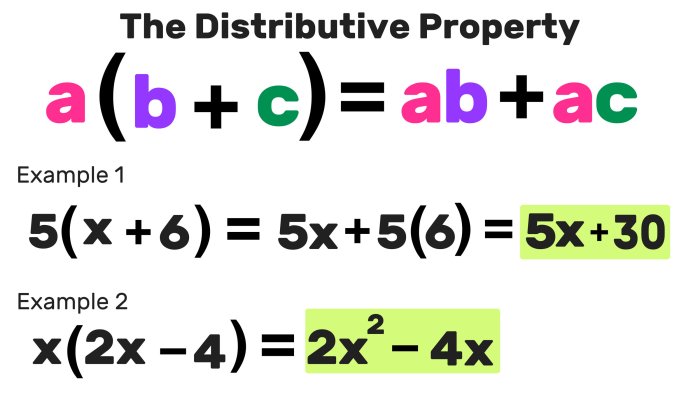
Multiplying polynomials is an essential algebraic operation that involves combining two or more polynomials to obtain a new polynomial. It is a fundamental skill in algebra 2 and is used in various applications, such as solving equations, factoring polynomials, and simplifying expressions.
There are several methods for multiplying polynomials, each with its own advantages and disadvantages. The most common methods are the horizontal method and the vertical method.
Methods for Multiplying Polynomials
Horizontal Method
The horizontal method, also known as the long multiplication method, is a straightforward and widely used method for multiplying polynomials. It involves multiplying each term of the first polynomial by each term of the second polynomial and then combining like terms.
For example, to multiply the polynomials (x + 2) and (x – 3), we would set up the multiplication as follows:
“`(x + 2)
- (x
- 3) =
“““x^2
- 3x + 2x
- 6 =
“““x^2
- x
- 6
“`
Vertical Method
The vertical method, also known as the box method, is another common method for multiplying polynomials. It involves arranging the polynomials vertically and multiplying each term of the first polynomial by each term of the second polynomial. The products are then added together to obtain the final result.
For example, to multiply the polynomials (x + 2) and (x – 3) using the vertical method, we would set up the multiplication as follows:
“` x
3
x + 2 | x^2
3x
+ 2x
6
“““ x^2
- x
- 6
“`
Special Products
In addition to the general methods for multiplying polynomials, there are several special products that can be used to simplify the multiplication of certain types of polynomials.
Multiplying Binomials
The special product for multiplying binomials is given by:
“`(a + b)
(c + d) = ac + ad + bc + bd
“`
For example, to multiply the binomials (x + 2) and (y – 3), we would use the special product as follows:
“`(x + 2)
- (y
- 3) = xy
- 3x + 2y
- 6
“`
Multiplying Trinomials
The special product for multiplying trinomials is given by:
“`(a + b + c)
(d + e + f) =
“““ad + ae + af + bd + be + bf + cd + ce + cf“`
For example, to multiply the trinomials (x + y + z) and (w + x + y), we would use the special product as follows:
“`(x + y + z)
(w + x + y) =
“““xw + x^2 + xy + yw + y^2 + yz + zw + zx + zy“`
Applications of Multiplying Polynomials, Multiplying polynomials algebra 2 worksheet
Multiplying polynomials has numerous applications in algebra 2, including:
Solving Equations
Multiplying polynomials can be used to solve equations by isolating the variable on one side of the equation and multiplying both sides by the same non-zero polynomial.
For example, to solve the equation x^2 – 5x + 6 = 0, we would multiply both sides by (x – 3) as follows:
“`(x
- 3)
- (x^2
- 5x + 6) = 0
“““x^3
- 8x^2 + 21x
- 18 = 0
“`
We can then solve the resulting cubic equation for x.
Factoring Polynomials
Multiplying polynomials can also be used to factor polynomials by finding two or more polynomials that, when multiplied together, give the original polynomial.
For example, to factor the polynomial x^2 – 5x + 6, we would find two binomials that, when multiplied together, give x^2 – 5x + 6. These binomials are (x – 2) and (x – 3), so we can write:
“`x^2
- 5x + 6 = (x
- 2)
- (x
- 3)
“`
FAQ Compilation
What are the different methods for multiplying polynomials?
The horizontal method and vertical method are two common methods for multiplying polynomials.
What are special products in polynomial multiplication?
Special products include multiplying binomials and trinomials, which have specific formulas and patterns.
How can multiplying polynomials be used in real-world applications?
Multiplying polynomials finds applications in solving equations, factoring polynomials, and modeling real-world scenarios.